Collecting evidence from observation and then using it to plan instruction is challenging work, particularly in preschool and early primary grades. Children frequently showcase their mathematical thinking using materials that will be moved around during learning and put away at the end of math activities, leaving their mathematical thinking undocumented. Teachers often use observation tools or checklists to record evidence of children’s thinking (Fennell, Kobett, & Wray 2017). Using these types of tools requires teachers to skillfully attend to and interpret a child’s work and talk, often in a moment filled with the buzz of a classroom full of young children (Jacobs et al. 2011).

One way to simplify teachers’ work of attending to and interpreting children’s thinking is to give teachers more time to analyze evidence. Capturing children’s thinking using photographs enables teachers to examine children’s work later on, when they have time to reflect on what children know and how children understand it. Furthermore, the photographs can be an effective starting point for the formative assessment cycle focused on assessment for learning (Wiliam 2017), which can be informed by learning progressions (Ebby & Petit 2018).

This article describes how two tools—photographs and learning trajectories—can be used to capture children’s mathematical thinking and reflect on it to make instructional decisions as part of the formative assessment process. To illustrate this, we consider the case of Ms. Lopez, a preservice teacher, as she uses these tools to document and make sense of children’s additive reasoning in a kindergarten math station.

Capturing children’s mathematical thinking with photographs

Documenting children’s understanding is a key element of formative assessment, and when it comes to mathematical thinking, the use of photographs offers many benefits. Photographs can give teachers and families visual evidence of children’s mathematical development over time (Krechevsky et al. 2013). In preschool and primary classrooms, the mathematical work of children is not always expressed in a written form; photographs capture children’s math activities with blocks, counters, games, and other materials (Tapper 2012). In addition, photographs provide teachers with evidence of children’s understandings that can be analyzed at a later time, away from the buzz of the classroom (Sherin & van Es 2003). The use of photographs makes it possible for teachers to make sense of children’s mathematical thinking outside of the classroom moment and to plan future math instruction more intentionally.

Understanding children’s progress with learning trajectories

Learning trajectories (Clements & Sarama 2014), or learning progressions (Ebby & Petit 2018), have been created to synthesize and reflect research and knowledge about how children learn and understand mathematics. Learning progressions provide teachers with a framework for interpreting children’s mathematical thinking and can be used when planning instruction to move children’s understandings forward (Ebby & Petit 2018). For example, the Ongoing Assessment Project (OGAP) Additive Framework displays the strategies children may use when counting and solving additive problems (OGAP Math LLC 2017). It also provides illustrations of student work that teachers can expect to see when children solve additive problems, beginning with counting strategies and moving toward more complex and generalizable strategies. Having an image of what children’s thinking might look like when they are working, supports teachers so they can (1) plan by anticipating children’s strategies and responses to a problem or task; (2) be prepared to carefully observe children so that their mathematical thinking and strategies are easier to identify and capture in the moment; (3) interpret children’s work; and (4) design instruction that builds on children’s current understandings and moves their mathematical thinking forward.

Ms. Lopez’s math station

Ms. Lopez, the preservice teacher, is taking an elementary mathematics methods course taught by the first author. An assignment in the instructional methods course is to create a math station (Diller 2011) for use as a formative assessment tool. The assignment asks preservice teachers to engage children in the math station, photograph evidence of the children’s mathematical thinking at the station, analyze the photographs, and consider potential next instructional steps based on those analyses. Ms. Lopez decided to complete the assignment in her field placement, a kindergarten classroom where she had been observing the teacher and the children.

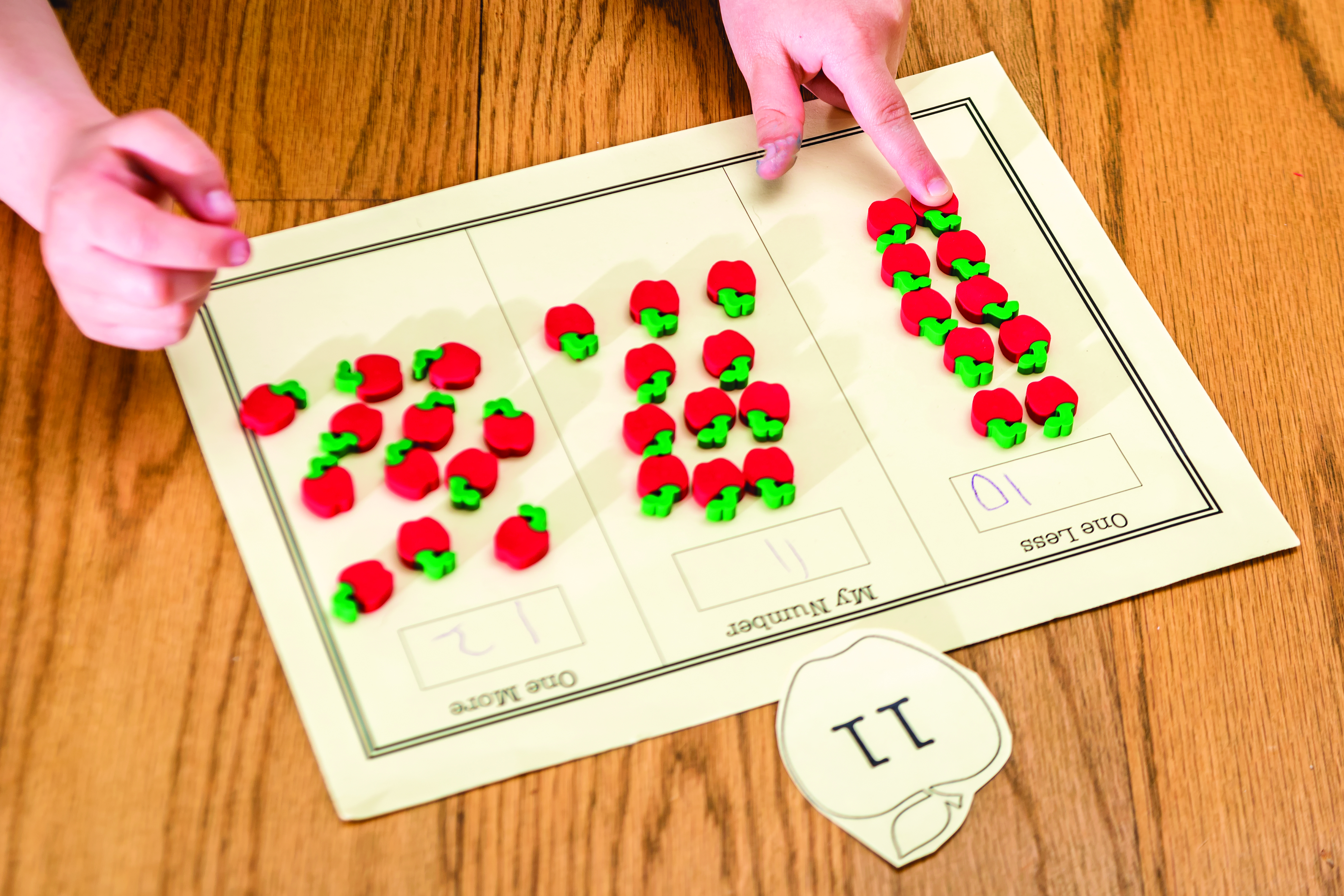
Ms. Lopez knew the children had been working on counting from 0 to 20, so she created her math station to extend the children’s mathematical experiences by meeting two instructional goals: (1) to identify a number from 0 and 20 and then build the numbers that are one more and one less than the original number, and (2) to practice writing numbers from 0 to 20 that represent a set of objects. In the math station, the children each selected a number from a deck of number cards and used apple-shaped erasers to create concrete representations of their numbers. Then the children determined which numbers were one more and one less and represented those values with erasers. Finally, on a recording sheet, the children wrote the numbers.

Before engaging children at the math station, Ms. Lopez spent time examining the OGAP Additive Framework to familiarize herself with the ways children might represent and count the selected quantities. Reviewing the framework helped her determine that the key objective of her formative assessments (and the photographs) would be to notice and document how children were counting. Specifically, she wanted to know if and how children organized or grouped the concrete objects. Ms. Lopez thought photographing this aspect of the children’s activities at the station was appropriate because once children left the math station, the concrete objects would be put away and the evidence of their thinking would no longer be available to inform her instructional planning.

Once all of the children completed the math station activity, Ms. Lopez looked at the photographs she had taken during the activity and selected a few to use for the course assignment. Here, we examine two of the photographs Ms. Lopez selected and discuss how each increased her understanding of the children’s mathematical thinking.

Ms. Lopez described the photograph above as follows: “[The photograph] provided an accurate representation of [how] two children chose to solve their number card using different strategies.” Examining the photo, which was taken mid-activity, she noted that Rosa (the student on the left) had begun organizing the apples by creating rows of four, whereas Jillian (the student on the right) was counting her apples by ones and had them all together in a single group. According to Ms. Lopez, Rosa’s organizational strategy was based on her previous knowledge: “Rosa preferred placing her counters in groups of four because she felt confident in her ability to count by fours. Rosa said her counting strategy reminded her of counting quarters. She knew that four quarters make a dollar, and that’s how she wanted to count.”

Rosa then used her organizational strategy to help Jillian. “Rosa explained to Jillian how she could group her counters into groups of five to help make her counting easier,” Ms. Lopez recalled. From her observation, Ms. Lopez noticed something else: “Jillian felt confident in her ability to count groups of fives, and this made it easier for her to identify one more and one less.”

The second photograph Ms. Lopez described (above) considered the strategy of skip counting but highlighted a student misconception. She wrote,

Ms. Lopez’s analyses of the photographs demonstrate her ability to attend to the mathematics in the photographs. Her analyses also highlight the power of having photographs to capture children’s thinking—including photographs of in-progress activities that show children’s thinking as it is happening rather than “finished products”—and to allow time for in-depth analyses later. Using her photographs, Ms. Lopez was able to specifically describe the strategies the children were using, whether grouping by fours or fives or counting by ones, and she was able to recognize the challenges Shannon experienced when trying to add twos. She was also able to describe and interpret the children’s strategies. She acknowledged that Rosa had an efficient strategy for counting while Jillian did not, and that Rosa’s strategy was illustrative and enabled the children to work together to advance Jillian’s counting strategies. Ms. Lopez’s use of photographs to capture and interpret children’s mathematical thinking and actions provided her with formative assessment data she could use to plan for further student learning.

After Ms. Lopez made sense of the children’s thinking that was visible in all of the photographs she took throughout the activity, she used the OGAP Additive Framework as a way of identifying and describing the children’s understandings, based on the images in the framework’s learning progressions. For example, Ms. Lopez stated, “Rosa’s strategy falls under the flexible counting section of the OGAP Framework because there is evidence of her counting by twos, fours, and fives, as well as identifying one more and one less.”

In the moment, while Rosa was engaged at the math station, Ms. Lopez had many responsibilities in the classroom. It would have been nearly impossible for her to spend the time or give the attention necessary to Rosa’s work in order to analyze it and determine where it belonged in the learning progressions. Taking photographs gave her the ability to analyze Rosa’s strategies thoroughly and accurately when she had more time.

Ms. Lopez also used the OGAP Additive Framework to figure out her next instructional steps. For example, she began by describing how Shannon’s counting strategy of adding 2 + 2 + 2 + 2 + 2 was evidence that Shannon’s work would be considered an early counting strategy. She went on to suggest the following instructional steps:

Using the OGAP Additive Framework to identify Shannon’s work along a developmental progression of additive reasoning, Ms. Lopez was able to suggest the instructional next step of “working with the teacher one-on-one to build her counting fluency.” Although this is not a sophisticated instructional suggestion, it is a thoughtful beginning step for a preservice teacher and a step that was made possible by the opportunity to examine the child’s thinking outside of the moment.

Another benefit of photographs is that they enable meaningful collaboration with colleagues outside the classroom. Ms. Lopez’s instructional methods professor challenged her thinking about how she could better connect the evidence in Shannon’s work to a more productive instructional response. Together, they used the photograph and the OGAP Additive Framework to construct an alternative plan for next instructional steps. In those next steps, they leveraged Shannon’s desire to use skip counting as a strategy and gave her concrete models to explore and practice skip counting.

The important idea to consider in this case is that planning for and collecting photographic evidence, coupled with the use of learning progressions, enabled Ms. Lopez to identify children’s mathematical thinking, make sense of it, label it along a developmental trajectory, and consider instructional next steps, all of which are key elements of the formative assessment cycle.

A call to action

This case illustrates how teachers can use photographs and learning trajectories as tools to support formative assessment practices and intentional instruction. The photographs provide a means of capturing evidence of children’s mathematical thinking that would otherwise have disappeared following the math activity. The visual structures of various additive strategies in the OGAP Additive Framework offer the teacher a lens through which to notice and identify important mathematical thinking to capture in photographs. Analyses of the photographs with the learning progressions further support the teacher as she interprets the thinking children are doing and identifies how to build on their thinking for future instruction. In this way, photographs serve as a vehicle for the formative assessment cycle.

We invite teachers to plan photographic opportunities in their classrooms and to try using photographs along with learning progressions as a way to capture and make meaning of formative assessment data that typically disappear during cleanup time. Similar to Ms. Lopez, we encourage teachers to select a few photographs to investigate in more detail. The following questions might be used to guide this investigation:

• What evidence of mathematical thinking is present in your photographs?
• Are any of the strategies children used identifiable in the learning progressions?
• What strategies do I want to encourage or build on in tomorrow’s instruction?

  ---

   

References

Clements, D.H., & J. Sarama. 2014. Learning and Teaching Early Math: The Learning Trajectories Approach. 2nd ed. Studies in Mathematical Thinking and Learning series. New York: Routledge.

Diller, D. 2011. Math Work Stations: Independent Learning You Can Count On, K–2. Portland, ME: Stenhouse; Markham, ONT: Pembroke.

Ebby, C., & M.M. Petit. 2018. “Using Learning Trajectories to Elicit, Interpret, and Respond to Student Thinking.” In A Fresh Look at Formative Assessment in Mathematics Teaching, eds. E.A. Silver & V.L. Mills, 81–102. Reston, VA: NCTM (National Council of Teachers of Mathematics).

Fennell, F., B.M. Kobett, & J. A. Wray. 2017. The Formative 5: Everyday Assessment Techniques for Every Math Classroom. Thousand Oaks, CA: Corwin; Reston, VA: NCTM.

Jacobs, V.R., L.L.C. Lamb, R.A. Philipp, & B.P. Schappelle. 2011. “Deciding How to Respond on the Basis of Children’s Understandings.” In Mathematics Teacher Noticing: Seeing Through Teachers’ Eyes, eds. M.G. Sherin, V.R. Jacobs, & R.A. Philipp, 97–116. New York: Routledge.

Krechevksy, M., B. Mardell, M. Rivard, & D. Wilson. 2013. Visible Learners: Promoting Reggio-Inspired Approaches in All Schools. San Francisco, CA: Jossey-Bass.

OGAP Math LLC. 2017. “OGAP Additive Framework.” www.ogapmath.com/wp-content/uploads/2017/04/framework_November2017.pdf.

Sherin, M.G., & E.A. van Es. 2003. “A New Lens on Teaching: Learning to Notice.” Mathematics Teaching in the Middle School 9 (2): 92–95.

Tapper, J. 2012. Solving for Why: Understanding, Assessing, and Teaching Students Who Struggle With Math, Grades K–8. Sausalito, CA: Math Solutions.

Wiliam, D. 2017. Embedded Formative Assessment. 2nd ed. Bloomington, IN: Solution Tree.